What I had done (err...sorry for the lack of extra graphics...):
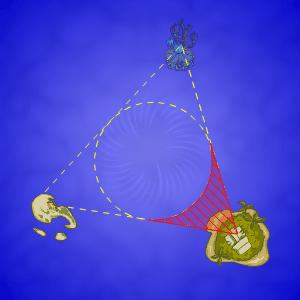
Let
M be the Mystery Island.
C be the centre of the circle. [ie the incentre of the large triangle]
K be the pt where the circle meets the line between M and Krawk Island
W be the pt where the circle meets the line between M and Maraqua
i)
congruent triangles:
CK = CW
(they are radii of the same circle)
angle CKM = angle CWM = 90 deg
(tangents...)
MC = MC
(obviously =.=)
Thus, we get triangle CKM congruent to triangle CWM
(a right angle, the hypotenuse, and a side/leg)
From this, we get:
area of CKM = area of CWM
i.e.
area of CWMK = 2*(area of CKM)
angle CMK = angle CMW
i.e.
angle KMW = 2*(angle CMK)
ii)
A certain angle: (or well...angles)
Using the data given in the previous LC:
By cosine law,
angle KMW = 80.406... deg
2*(angle CMK) = 80.406... deg (by (i))
angle CMK = 40.203... deg
also,
sum of interior angles of CWKM = 360 deg
angle WCK = (360 - 90 - 90 - 80.406) deg
angle WCK = 99.594... deg
iii)
radius:
From the previous LC,
radius = 76.064... km,
which includes
CK.
Also remember that
angle CKM = 90 deg
Thus, by the definition of tangent,
CK / KM = tan(angle CMK) (by (ii))
KM = 90.000... km
iv)
area:
area of CKM = CK * KM /2
2*(area of CKM) = CK * KM
area of CWMK = CK * KM =
6846... sqkm
area of circle = pi * (radius)^2 = 18176... sqkm
area of sector CWK = (area of circle) * (angle WCK)/(360 deg),
which is
5028... sqkm
req. area = (area of CWMK) - (area of sector CWK),
which is 1818... sqkm.
Hence, rounding up, we would get 1825 sqkm.



